lukenlow
2018-06-22 12:27:08 | Excellent info, it is very useful to learn such things in trivial discussions 192.168.O.1 |  |
hooverbm
2012-11-01 12:24:49 | A quick way I did this is similar to chemicalsoul's solution.
The wavelength is easy to pick off from the graph, 1cm. We're given the velocity.
v = wavelength*frequency.
f = 500 Hz
This is a beat frequency, thus the difference in frequency between the two oscillations must be very close to 500 Hz.
Choice D is the only solution that satisfies that condition. |  |
len x 1624
2012-04-16 20:10:16 | Simple Solution:rnrnCount the number of times the small wave occurs within the large wave. For every one large wave there exist six small waves; therefore the frequency of the small wave is 6x the frequency of the large wave. The only instance where the smaller wave has a frequency 6x larger is in choice (D). rnrnChoice (B) is incorrect because the larger wave (3 Volts) has the higher frequency.
aprilrussell
2018-05-30 03:45:18 |
A quick way I did this is similar to chemicalsoul\'s solution. https://run2.online/run-3 Thanks
|
|  |
cathaychris
2011-10-13 21:26:53 | Since the higher frequency oscillation is easy to pick out, note that one period is about 1 cm at .5 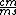 = 2 ms....... = 2 ms....... 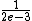 = 500 Hz, which only appears in one place, (D). You can further reassure yourself by noting the high-frequency amplitude is about 1.25 cm, pk-pk * 2 V/cm = 2.5 = 500 Hz, which only appears in one place, (D). You can further reassure yourself by noting the high-frequency amplitude is about 1.25 cm, pk-pk * 2 V/cm = 2.5 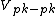 . Remember amplitude is half of the pk-pk value. . Remember amplitude is half of the pk-pk value. |  |
mrTrig
2010-10-23 12:42:33 | ****First line typo****
time is missing on left side. should be
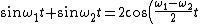}\sin{\left(\frac{\omega_{1}+\omega_{2}}{2}t\right)}) |  |
chemicalsoul
2009-10-17 01:50:33 | From the given graph, we can approximate that the wavelength is 1cm and the amplitude is .5 cm=1v. Therefore, the frequency diff between the two waves should be near to 500 Hz and the amp diff should be about 1 v. (D) is the closest ! |  |
ravenseldon666
2008-10-25 00:36:44 | the waves are of different amplitudes so how come they add up to the form product of two sines of a frequency sum and frequency difference? |  |
dumbguy
2007-10-16 11:05:44 | Don't forget the scales of the oscilliscope. x is equivilant to freq. while y is equivilant to volts. You'll notice that the period of the the higher freq. wave is about 1 cm.( i used the bottoms of the first two) Since it is given that .5cm/ms, you can deduce that 1 cm is 2 ms. T=1/f, so the period is 1000/2 or 500hz. Choice D is the only one that has this. If not coninved, then check the amplitude. The amplitude is a little more then .5cm, which means it has to be a little more then 1 volt, which fits with D and its freq. as well. If you do the same thing for the wave envolope, you will get a freg. less then 100, around 80 and an amplitude of 2.5 volts. Don't forget when drawing the wave envolope to go thru the center of the higher freq. waves. |  |
adam
2005-11-09 22:25:22 | From hind sight...
If you count the periods for the high freq that occur during the 1 period of the lower freq you will get a ration about 6:1. This means that 6(low freq)= high freq. This satisfies B and D. But B has the amplitudes wrong (higher freq should have a smaller amplitude than low freq) leaving D as the answer.
flyboy621
2010-10-23 08:02:38 |
This is the way to do it. The smaller wave has 6 times the frequency and half the amplitude of the larger. Choice (D) is the best.
Good ol' hindsight...
|
|  |
