GREPhysics.NET: GR9277 #99

GR9277 #99

Problem

GREPhysics.NET Official Solution

\prob{99}
In perturbation theory, what is the first order correction to the energy of a hydrogen atom (Bohr radius $a_0$ ) in its ground state due to the presence of a static electric field E?

0
$eEa_0$
$3eEa_0$
$\frac{8e^2Ea_0^3}{3}$
$\frac{8e^2E^2a_0^3}{3}$

Quantum Mechanics $\Rightarrow$ }Perturbation Theory

The perturbed Hamiltonian is given by $\Delta H = eEz = eEr\cos\theta$ , where the last substitution is made for spherical coordinates.

The first-order energy-shift is given by $\langle \psi_0 | \Delta H | \psi_0 \rangle$ , where $\psi_0\propto e^{-kr}$ .

$dV=r^2drd\Omega=r^2\sin^2\theta d\phi d\theta dr$ .

Thus, $E_0 = \int_0^\infty \int_0^{\pi} \int_0^{2\pi} e^{-kr}\cos\theta\sin^2\theta d\theta d\phi =0$ , since the integral of $\cos\theta\sin^2\theta$ over $0$ to $\pi$ is 0.

Search this site for keyword perturbation for more on this.

Alternate Solutions

marching_band_to_nowhere 2019-09-02 22:42:59	This is a question about the Stark Effect, which is known to not affect the ground state electrons in any orbital. Thus, we know the first order correction to the energy of the ground state is 0 by definition. Reply to this comment
evanb 2008-06-27 14:46:04	The wave function of 1s is even, the perturbative operator = - q * Efield * Z is odd. Thus the first-order matrix element must be zero. Reply to this comment

Comments

marching_band_to_nowhere
2019-09-02 22:42:59

This is a question about the Stark Effect, which is known to not affect the ground state electrons in any orbital. Thus, we know the first order correction to the energy of the ground state is 0 by definition.

Reply to this comment

marching_band_to_nowhere
2019-09-02 22:42:44

Reply to this comment

enterprise
2018-03-31 21:36:41

In the ground state , The wavefunction is rotationally invariant. An Electric field points at some definite direction. The energy will depend on the angle between the Electric field and the dipole moment of the hydrogen. Obviously this dipole can\'t point at any definite direction because our atom is in a ground state. So , there shouldn\'t be any corrrection to the energy. Corrections should occur to P states because they have Y(cos(\\theta)) dependence in their wave functions. Otherwise , you will get $\\int_{-1}^{+1}dcos(\\theta) cos(\\theta)=0$ if there is no other factors in the integrand to prevent it from being an odd function in cos(theta)

Reply to this comment

bagel
2011-11-11 19:46:02

No math necessary:

Bohr's energy calculation in a hydrogen atom was calculated solely on the presence of E field. QED.

liliapunto
2013-07-16 02:41:54

yep. solvable system. no correction.

James6M
2014-10-17 21:28:23

I think that the question is referring to an external (and uniform) electric field. However, the question fails to make that clear.

Reply to this comment

keradeek
2011-10-11 23:07:06

V ' = V0 * z

The first order correction to the energy is the expectation value of the perturbing Hamiltonian. In other words, it's the expectation value of V0*z, or
V0*.

The ground state is symmetric in z. = O

Answer is A.

keradeek
2011-10-11 23:09:44

The site does not allow me to use brackets. Sorry.

The answer is equal to the expectation value of V0*z. The expectation value of z is zero. Answer is A

Reply to this comment

evanb
2008-06-27 14:46:04

The wave function of 1s is even, the perturbative operator = - q * Efield * Z is odd.

Thus the first-order matrix element must be zero.

nyuko
2009-10-30 23:05:54

I want to post the same thing as you did. But you are one year ahead of me :)

Reply to this comment

zzzzort
2007-11-01 14:26:58

None of the other answers have the correct units.

jburkart
2007-11-02 00:48:27

No, electric field times charge is force, and force times distance is work, a form of energy. You have to do it the proper way.

Reply to this comment

herrphysik
2006-09-14 23:08:00

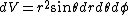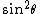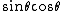Minor typo: In spherical coordinates $dV = r^2 sin{\theta} dr d \theta d \phi$ (not $sin^2{\theta}$ ) and the limits for theta are 0 to 2*pi. And of course the integral of $sin{\theta} cos{\theta}$ from 0 to 2*pi is 0.

E^2Pi-1=0
2006-11-30 19:34:06

The limit is still 0 to pi ... theta is azimuthal angle.... but integral of Sin[theta]Cos[theta] from 0 to pi is still zero.....

p_e_duffy
2013-05-25 08:45:43

theta is the polar angle

Reply to this comment

Post A Comment!

You are replying to:

No math necessary: Bohr's energy calculation in a hydrogen atom was calculated solely on the presence of E field. QED.

Bare Basic LaTeX Rosetta Stone
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces $\alpha^2_0$ .
type this...	to get...
$\int_0^\infty$	$\int_0^\infty$
$\partial$	$\partial$
$\Rightarrow$	$\Rightarrow$
$\ddot{x},\dot{x}$	$\ddot{x},\dot{x}$
$\sqrt{z}$	$\sqrt{z}$
$\langle my \rangle$	$\langle my \rangle$
$\left( abacadabra \right)_{me}$	$\left( abacadabra \right)_{me}$
$\vec{E}$	$\vec{E}$
$\frac{a}{b}$	$\frac{a}{b}$

The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it...

All-Solutions List | Latest Comments | Unanswered Questions (Cries for Help!) |::| About

This site is not affiliated with ETS, nor is it officially endorsed (yet) by ETS. This site is the work of an individual named Yosun Chang. The solutions, sans user comments and ETS questions, are © 2005 by Yosun Chang except where noted. All rights reserved. / The comments appending particular solutions are due to the respective users. / Educational Testing Service, ETS, the ETS logo, Graduate Record Examinations, and GRE are registered trademarks of Educational Testing Service. The examination questions are © 1997 and © 2001 by ETS.

Bare Basic LaTeX Rosetta Stone