casseverhart13
2019-09-13 07:54:17 | Simply desire to say your problem is amazing. click here |  |
ernest21
2019-08-10 03:09:53 | I see the answers but I cannot find the questions. Where are they? There was a \"jump into the question\" buton in the old site that I can\'t see now. pixelmon island |  |
joshuaprice153
2019-08-08 05:56:52 | I’ve been visiting your blog for a while now and I always find a gem in your new posts. Thanks for your usual wonderful effort. towing service |  |
timfinnigan
2018-05-19 03:40:44 | Simple Work-KE Thm:\r\n\r\n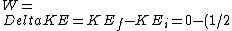(5)(10)^2 =-250) \r\n\r\n \r\n\r\n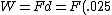) \r\n\r\n \r\n\r\n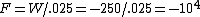 \r\n\r\nThe magnitude of the force, \r\n\r\nThe magnitude of the force, 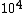 , is the answer (the negative could be thought of as direction). , is the answer (the negative could be thought of as direction). |  |
arashtavassoli
2016-10-17 15:35:39 | I think this question is somehow vague. Actually the time average and position average are not generally the same. But this question says just average force and it is not clear which average it means. However, the given information of the question is adequate only for calculation of position average which is 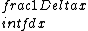 . I don\'t see any way to calculate time average of the force which is . I don\'t see any way to calculate time average of the force which is 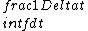 by using the given information of this question. by using the given information of this question. |  |
abc111
2011-01-05 23:57:38 | strange!! why we are neglecting potential energy
pam d
2011-09-23 19:32:49 |
Your zero potential energy ought to be the surface of the wood. If you are concerned about the change in gravitational potential energy of the nail since it travels downward, understand that it is negligible. You would need a ridiculously huge nail for it to affect the answer. Also note that the question asks for what the average force "most nearly" is.
|
|  |
Kabuto Yakushi
2010-09-02 09:25:45 | Just use the Impulse-Momentum theorem.
F 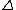 t = M t = M 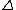 V V
the answer falls out. |  |
rlorek
2010-07-30 13:18:33 | Here's my bizarre method using the chain rule.
Force=dp/dt
since this problem describes a change in position instead of a change in time I used the chain rule to describe the above equation in terms of dx.
Force=dp/dt * dx/dx = dp/dx * dx/dt
p= mass * velocity = 5.0kg * 10m/s = 50kgm/s
dp/dx = (50kgm/s) / (0.025 m) = 2000 kg/s
dx/dt = the final velocity = 10m/s
dp/dx * dx/dt = 2,000kg/s * 10m/s = 20,000kgm/s^2 = 20,000N
But this question asked for the average force (Fa)!
Fa = (Force final + Force initial)/2
Force initial is the Force on initial impact (20,000N) and Force final is the force exerted immediately after the nail stops moving (0N). so....
Fa = (20,000N + 0N)/2 = 10,000N
By this method I come up with answer "D". |  |
shafatmubin
2009-11-03 09:14:39 | For the ones who have worked rigorously with equations of motion and/or tutored high school physics:
v^2 = u^2 + 2ax
=> 10^2 = 2a (0.025)
=> a = 100/0.05 m/s/s
After that, F = ma = 10^4 N
|  |
minaesm
2009-10-05 23:16:41 | we can solve the problem using the method of conservation of energy : 0.5 m v^2=mgy+V
V is the work done by the average force.
V=250-1.25 so V~250.
F=dV/dx=250/0.025=10000N |  |
dcn
2008-03-26 09:21:36 | Correct me if I'm wrong but isn't Average Force defined simply as delta p/delta t. Since the stone comes to rest, assuming all momentum is transfered to the nail, the momentum of the stone just before it hits the nail is delta p. For delta t we can use the average v which is (10-0)/2 = 5m/s. Then
delta t = y/(average v) =0.025/5.
Plugging this in:
Average F = (5kg)(10m/s)(5m/s)/(25x10^-3m)=10^4N.
Imperate
2008-09-11 12:23:00 |
This is the way I did it.rnIf the block has momentum 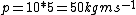 Then this momentum must be reduced to zero over the course of the nail being driven in. The time it the nail travels can be found from Then this momentum must be reduced to zero over the course of the nail being driven in. The time it the nail travels can be found from  Which implies Which implies 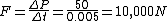 rnrnSo it can be done this way, but I wish I thought of using energy at the time. rnrnSo it can be done this way, but I wish I thought of using energy at the time.
|
Setareh
2011-10-24 00:30:23 |
I doubt whether you are allowed to use this equation : t=y/v or not, since we don't deal with constant speed motion, actually we have acceleration, so this equation is not valid in this case.
|
kronotsky
2018-10-23 02:46:41 |
This solution is essentially the same as the one given, because in both cases the assumption is made that the nail experiences a constant force. This is the correct approach for the time average of the force, but we still need to know the time dependence. Similarly, conservation of energy is the right approach to find the distance average of the force.
|
|  |
Richard
2007-10-31 12:36:30 | I think it would be better phrased as a Work Problem. It's not really energy conservation, because energy is clearly not conserved.
Anyway, not that it makes any difference to the solution really, but you forgot to include the potential energy term 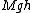 . It only contributes 50 J or so...so who cares right? . It only contributes 50 J or so...so who cares right?
|  |
kicksp
2007-10-29 09:33:05 | I have to disagree with everyone. Kinematics give the average net force as 100000/9.81 N 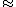 10190 N (downward), but the question asks for the average net force exerted BY the stone on the nail. (The binomial theorem will be useful to make this approximation). The average frictional force acting on the nail is 10190 N (downward), but the question asks for the average net force exerted BY the stone on the nail. (The binomial theorem will be useful to make this approximation). The average frictional force acting on the nail is 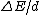 = 10000N (upward) from the expression for the mechanical energy lost due to a constant dissipative force. The magnitude of the force exerted on the nail BY the stone is therefore approximately 190 N. As an order of magnitude answer, then, the best anwer is B. = 10000N (upward) from the expression for the mechanical energy lost due to a constant dissipative force. The magnitude of the force exerted on the nail BY the stone is therefore approximately 190 N. As an order of magnitude answer, then, the best anwer is B.
Go maroons!
kicksp
2007-10-29 13:05:19 |
Sorry, I read the problem wrong. the average force exerted ON the stone BY the nail is 10000 N:
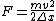
By Newton's Third Law, this is the magnitude of the average force exerted ON the nail BY the stone. Still, the analysis by energy conservation is erroneous.
|
kicksp
2007-10-29 13:07:18 |
Using the work-energy theorem is more appropriate.
|
|  |
kicksp
2007-10-29 09:32:30 | I have to disagree with everyone. Kinematics give the average net force as 100000/9.81 N 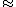 10190 N (downward), but the question asks for the average net force exerted BY the stone on the nail. (The binomial theorem will be useful to make this approximation). The average frictional force acting on the nail is 10190 N (downward), but the question asks for the average net force exerted BY the stone on the nail. (The binomial theorem will be useful to make this approximation). The average frictional force acting on the nail is 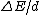 = 10000N (upward) from the expression for the mechanical energy lost due to a constant dissipative force. The magnitude of the force exerted on the nail BY the stone is therefore approximately 190 N. As an order of magnitude answer, then, the best anwer is B. = 10000N (upward) from the expression for the mechanical energy lost due to a constant dissipative force. The magnitude of the force exerted on the nail BY the stone is therefore approximately 190 N. As an order of magnitude answer, then, the best anwer is B.
Go maroons!
|  |
KarstenChu
2007-04-01 00:00:39 | Respectfully, I must argue these solutions.
First of all, I do not believe we can apply conservation of energy to this problem. It's possible that its just really late and I am really tired, but it seems to me that energy isn't necessarily conserved here since friction is slowing down the nail and dissipating energy in heat and sound. Additionally, the problem does not say that the collision between the stone and the nail is elastic so I see no reason to attack this problem from a conservation of energy foundation.
I do believe that conservation of momentum, though, is the way to go. I used two equations in my solution, x=v0t-1/2at^2 and J = Ft = delta p = m delta v.
Given that x = .025, delta v = 10 = v0 and F=ma, I solved for t in the impulse equation and plugged into the kinematic eqn to solve for F.
hungrychemist
2007-08-26 19:18:40 |
This question cannot be solved exactly. Your kinematic approach is only approximation since one cannot assume the constant acceleration of the pin. Like Yosun said, energy conservation approach is the way to go although energy is not really conserved here you can see from the choices, within a reasonable loss of energy due to (friction and sound and so on) you can conclude the ans to be D.
|
TigerTed8
2007-09-17 19:36:41 |
Karsten, I did the problem the same way as you when I first approached it. However, I'm now a convert to the energy method. The friction of the nail, clearly non-trivial, is not a part of the equation here. The stone has energy, and then it loses that energy. This occurs primarily through some force. So 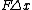 = = 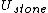 . The only way that the stone could lose energy that wasn't related to a force would be IR radiation, or the sound or heat from the stone on nail collision. The nail-wood friction occurs AFTER the transfer of energy from stone to nail by way of . The only way that the stone could lose energy that wasn't related to a force would be IR radiation, or the sound or heat from the stone on nail collision. The nail-wood friction occurs AFTER the transfer of energy from stone to nail by way of 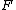 . Hope this helps. . Hope this helps.
|
kronotsky
2018-10-23 02:49:28 |
Who cares if energy is conserved? The energy lost by the nail is due to the forces on it.
|
|  |
jcain6
2005-12-09 05:13:08 | typo - below should read
F = ma = (5kg)(2000 m/s^2) = 10000 |  |
jcain6
2005-12-09 05:12:15 | you can also use kinematics here.
Use the velocity/position kinematic to solve for a:
a = 2000 m/s^2
where you use 0.025m as change in y.
F = ma = (5kg)(200m/s^2)= 10000N |  |
Ben
2005-12-02 11:19:26 | I think m was mass not meter, otherwise it made no sense at all. |  |
Ben
2005-12-01 13:11:39 | Should it not be 100m/2? As written, the answer would be 1,000 N...
daschaich
2005-12-01 21:45:21 |
Actually, it should be 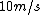 (dimensions and all that). Just a simple typo. Note that v is the initial speed and not the average speed. With (dimensions and all that). Just a simple typo. Note that v is the initial speed and not the average speed. With  ( (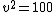 ), the equation becomes ), the equation becomes 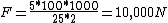 . .
|
yosun
2005-12-02 01:10:22 |
ben, daschaich: thanks for the typo-alert; it's been fixed now. (note to self: always ignore dimensions when doing the numerical calculations.)
|
|  |
