|
GR9677 #2
|
|
|
|
|
Alternate Solutions |
| There are no Alternate Solutions for this problem. Be the first to post one! |
|
|
Comments |
russian
2017-09-08 12:35:27 | Shortcut to avoid calculations. I noted that the induced current and the current due to the battery flow in opposite directions. The numerical values in this problem are such that it is easy to see that (A) is a trap answer for only finding the induced current and (C) is a trap answer for only finding the current due to the battery. Since the resulting current has to be less than the current due to the battery, choose (B). |  | cjohnson415
2013-06-23 14:44:53 | I really think that this solution is incorrect (as is the answer key in the physics GRE). The battery alone produces a current that flows clockwise. Current flows in the direction of decreasing potential because positive charges roll "downhill" in terms of potential differences.
Nature abhors a change in flux, so the induced voltage will produce a current which will produce a magnetic field into the page. To produce a magnetic field into the page, by the right-hand-rule, the current must flow clockwise, i.e. in the same direction as that caused by the battery.
Thus the battery voltage and the induced voltage add, yielding a total current of .65 A.
maxdp
2013-09-24 13:24:12 |
You're close, but your only confusion comes from thinking that the battery produces a clockwise current. The current always starts at the "big line" side of the battery (on the right), flows around the circuit (in this case counterclockwise) and ends at the "small line" side.
|
|  | bostroem
2013-03-24 08:19:41 | I think you're missing an integral sign on the RHS of Stoke's theorem. |  | phoxdie
2010-11-08 08:51:45 | These solutions are much more comprehensive than my own, but for anyone who might think like me.
I started by looking at the basic circuit to find
V=IR --> I=5/10=.5[A]
At this point I could only recall that nature abhors a change in flux, so using the RHR I could determine the direction of the induced current. In this case clockwise, which opposes the current produced by the 5V battery and 10 ohm resistor. I could not recall the relationship between flux and area to determine induced EMF, but it was clear that the solution must be < 0.5
Based on the geometry of the problem and the values given I assumed the answer should look like this
.5-x=.35 or .15
(the .35 and .15 come from answers A and B given).
If you rewrite this you find,
.5-.35 = x = .15 or .5-.15 = x = .35
Going back to the simple values given in the problem I could guess the answer should be the first of these equations, since the flux is equal to 150 and the only other terms in the problem are factors of 10. So I arrived at the answer B, which turns out to be right. Not sure if this logic is fool proof, but it at least helps when your mind goes blank on an easy problem. |  | caffeinated
2008-04-09 15:42:45 | By the way Yosun, thanks so much for sharing your knowledge with the rest of us. You've really helped me a lot. |  | caffeinated
2008-04-09 14:09:33 | The induced EMF opposes changes in current and not the constant dc current that is already there. The rate of change in B is negative. This flips the sign on the induced EMF.
caffeinated
2008-04-09 15:15:48 |
I have just encountered a useful explanation in REA's Physics Problem Solver, Problem 736 on page 721. According to these folks, the direction of the induced current opposes the change in the flux that causes the current in the first place. Since B is decreasing, the induced field is actually in the same direction as B. Pointing the thumb of the right hand in the direction of the induced field, the fingers point in the direction of the induced current, which is clockwise. This has nothing to do with the direction of the current from the DC voltage supply. It just happens to be in the opposite direction. In other words , if the voltage supply is flipped then the currents add. Incidentally, the B field is coming from somewhere else. It is not being caused by the current in the loop, and while there is a small field from the current in the loop, it is negligable compared to the whopping B>>dB=150T/s.
|
|  | Richard
2007-09-23 10:39:26 | The source alone produces a current, ![5[V]/10[\Omega]=.5[A]](/cgi-bin/mimetex.cgi?5[V]/10[\Omega]=.5[A]) clockwise. clockwise.
The change in the magnetic flux 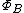 induces a current, induces a current, /10[\Omega]=.15[A]](/cgi-bin/mimetex.cgi?150[T/s](.01[m^2])/10[\Omega]=.15[A]) counterclockwise. counterclockwise.
The total current is then, ![.5[A]-.15[A]=.35[A]](/cgi-bin/mimetex.cgi?.5[A]-.15[A]=.35[A])
ewhite2
2007-10-24 09:55:55 |
Remember current flow is opposite the direction of electron flow; it is the flow of 'positive' charges. So the current from the battery flows counterclockwise. Also, since the magnetic field is decreasing in magnitude the induced current will flow clockwise (nature abhors a change in flux).
So the induced current does in fact oppose the current from the battery, however I believe your directions are mixed up.
|
|  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
