|
GR9277 #45
|
|
|
|
|
Alternate Solutions |
QuantumCat
2014-09-01 12:35:26 | The first thing I noticed was that it lost 64% of its kinetic energy, so it will only rise to 64% of it's original height. Even though it's a bit of a rehash from everything that has been mentioned, this solution solves the problem in just a few seconds. |  |
|
|
Comments |
QuantumCat
2014-09-01 12:35:26 | The first thing I noticed was that it lost 64% of its kinetic energy, so it will only rise to 64% of it's original height. Even though it's a bit of a rehash from everything that has been mentioned, this solution solves the problem in just a few seconds.
ETScustomer
2017-09-22 20:06:10 |
It kept* 64 percent of its kinetic energy.
|
|  | myscifilullaby
2012-04-03 13:59:07 | quickest way. Use ratios while also noting matweiss's comment:
h/(v^2)=x/((0.8v)^2)
x=(0.8^2)h=0.64h |  | matweiss
2010-11-13 18:11:12 | This is something everyone should know:
The height that an object rises to is proportional to the square of V/V(initial)
Example: if ball A is thrown up in the air at v= 100 m/s and ball B is thrown up in the air at 10 m/s what is the ratio of the height ball A will reach to the height ball B will reach.
the answer is (100/10)^2 = 100. Aka, ball A will go 100 times as high as ball B. If you don't believe me you can do the math out yourself, if you do, hopefully it'll be the last time you work it out this way. |  | ramparts
2009-08-09 20:45:30 | The quicker way to do this is to work entirely with energies (just skips a couple of steps):
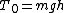 is the kinetic energy at the ground. Some amount of kinetic energy is lost there - since is the kinetic energy at the ground. Some amount of kinetic energy is lost there - since 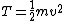 , we have , we have 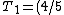^2 T_0) . (4/5)^2 is .64, so 36% of the energy is lost. So by conservation of energy again, with y as the height the ball reaches, . (4/5)^2 is .64, so 36% of the energy is lost. So by conservation of energy again, with y as the height the ball reaches, 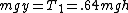 . . 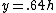 . Simple as that ;) . Simple as that ;) |  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
