GREPhysics.NET: GR9277 #41

GR9277 #41

Problem

GREPhysics.NET Official Solution

\prob{41}
A cylinder with moment of inertia $4kgm^2$ about a fixed axis initially rotates at 80 radians per second about this axis. A constant torque is applied to slow it down to 40 radians per second.

The kinetic energy lost by the cylinder is

80 J
800 J
4000 J
9600 J
19,200 J

Mechanics $\Rightarrow$ }Energy

The kinetic energy is related to the inertia I and angular velocity $\omega$ by $K = \frac{1}{2}I\omega^2$ .

The problem supplies $I=4kgm^2$ so one needs not calculate the moment of inertia. The angular velocity starts at $80 rad/s$ and ends at $40 rad/s$ .

Thus, the kinetic energy lost $\Delta K = \frac{1}{2}I(\omega_f^2-\omega_0^2)=\frac{1}{2}(4)(40^2-80^2)=2(1600-6400)=-9600J$ , as in choice (D).

Alternate Solutions

Ryry013
2019-10-06 09:10:04

A slightly faster way to do this problem is to know that if omega halves, then kinetic energy will decrease by 1/4. Then energy LOST is just (0.5)(4)(80^2) * (3/4) = (0.5)(6400)(3/4) = 9600J

Reply to this comment

Comments

Ryry013
2019-10-06 09:10:04

A slightly faster way to do this problem is to know that if omega halves, then kinetic energy will decrease by 1/4. Then energy LOST is just (0.5)(4)(80^2) * (3/4) = (0.5)(6400)(3/4) = 9600J

Reply to this comment

mnky9800n
2013-09-24 13:05:47

I also like to think in terms of the potential energy of a 100kg person who is about to fall 10 meters. That is mgh~(100 kg)(10 m/s^2)(10 m) = 10000 J. Thus A and B are probably too small and E is probably too big.

Reply to this comment

aloha
2008-10-10 03:21:51

Maybe it's a silly question, but how can we know that I=4?
Thanks :)

Monk
2008-10-13 20:11:46

it tells you in the question...it is 4 kg m^2

kg m^2 is the units not a formula

tensordyne
2008-11-04 13:16:28

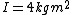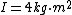I am with Monk, from reading the problem it seems like they are saying $I = 4 k g m^2$ is a formula in k (proportionality factor maybe), g ( $9.8 m/s$ ) and mass m instead of as units because there is no grouping of the units, such as in say $I = 4 kg \cdot m^2$ , which would have made the use of units much more manifest.

Reply to this comment

Post A Comment!

Bare Basic LaTeX Rosetta Stone
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces $\alpha^2_0$ .
type this...	to get...
$\int_0^\infty$	$\int_0^\infty$
$\partial$	$\partial$
$\Rightarrow$	$\Rightarrow$
$\ddot{x},\dot{x}$	$\ddot{x},\dot{x}$
$\sqrt{z}$	$\sqrt{z}$
$\langle my \rangle$	$\langle my \rangle$
$\left( abacadabra \right)_{me}$	$\left( abacadabra \right)_{me}$
$\vec{E}$	$\vec{E}$
$\frac{a}{b}$	$\frac{a}{b}$

The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it...

All-Solutions List | Latest Comments | Unanswered Questions (Cries for Help!) |::| About

This site is not affiliated with ETS, nor is it officially endorsed (yet) by ETS. This site is the work of an individual named Yosun Chang. The solutions, sans user comments and ETS questions, are © 2005 by Yosun Chang except where noted. All rights reserved. / The comments appending particular solutions are due to the respective users. / Educational Testing Service, ETS, the ETS logo, Graduate Record Examinations, and GRE are registered trademarks of Educational Testing Service. The examination questions are © 1997 and © 2001 by ETS.

Bare Basic LaTeX Rosetta Stone