|
GR9277 #19
|
|
|
|
|
Alternate Solutions |
wahoo1
2011-10-31 11:55:12 | you can also solve it pretty easily using the fact that g~10, G=6.67x10^-11.
g= Me G / Re^2 >> Me= gRe^2/G
using order of magnitudes: Me~ (10)(10^6)^2 / (10^-11)
this becomes (10^1)(10^12)(10^11)= 10^24 which is closest to choice (A). |  | dinoco
2010-11-07 06:23:30 | You can use 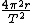 = = 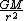 noting that a day is 86400 seconds and solve for M. noting that a day is 86400 seconds and solve for M. |  | SlickAce21
2005-11-11 11:05:34 | This problem is pretty easily solved by a usage of Kepler's law, if one remembers that g = (GM)/R^2, then one gets 10 = (6E-11)M/36E12, multiplying through you have M = 60E23 or 6E24 |  |
|
|
Comments |
justacomment
2014-09-30 16:51:36 | If you can't think of anything but the volume of a sphere (like myself), this is how I approach the answer:
You are given the radius of the Earth, so do the math then one shall find V~10^21 (m^3), using 2^10~10^3 as approximation.
Then remember the density of most elements on Earth lies in the scale of 10^3 (kg/m^3), and there you go, A is the only possible answer. |  | TMFMende
2012-10-26 23:10:20 | If you apply Gauss's law to gravity... you will know that
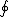 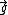  d d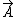 = 4 = 4  GM GM
or rather
g 4  r r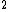 = 4 = 4  GM GM
ETS gives you r in the problem and G at the beginning of the test; you already know g (~10 m/s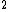 ). So solve for M: ). So solve for M:
M = gr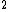 /G /G
...Gauss law will also be helpful if you need to calculate the force of gravity inside a mass (e.g. if there were a magical shaft going toward the center of the Earth in which you could send a test mass).
Thank you Yosun so much for this site! It's great to see the different ways people go about tackling the same problem. |  | wahoo1
2011-10-31 11:55:12 | you can also solve it pretty easily using the fact that g~10, G=6.67x10^-11.
g= Me G / Re^2 >> Me= gRe^2/G
using order of magnitudes: Me~ (10)(10^6)^2 / (10^-11)
this becomes (10^1)(10^12)(10^11)= 10^24 which is closest to choice (A). |  | wahoo1
2011-10-31 11:54:50 | you can also solve it pretty easily using the fact that g~10, G=6.67x10^-11.
g= Me G / Re^2 >> Me= gRe^2/G
using order of magnitudes: Me~ (10)(10^6)^2 / (10^-11)
this becomes (10^1)(10^12)(10^11)= 10^24 which is closest to choice (A). |  | dinoco
2010-11-07 06:23:30 | You can use 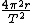 = = 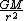 noting that a day is 86400 seconds and solve for M. noting that a day is 86400 seconds and solve for M.
The Bridge
2018-10-26 19:15:17 |
That won\'t work. The formula on the left is for centripetal acceleration of someone standing on the Equator due to the rotation of the Earth, and works out to about .88 m/s2. This is in fact many times less than 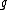 , the acceleration due to gravity of something on the Earth\'s surface, which is the right side. If you knew the orbital period of an object at the Earth\'s surface off the top of your head (84 min), then this method would work, but it gives you , the acceleration due to gravity of something on the Earth\'s surface, which is the right side. If you knew the orbital period of an object at the Earth\'s surface off the top of your head (84 min), then this method would work, but it gives you  10 m/s2 in the table of information at the top of the test anyway, so the left side of the equation is completely unneeded. 10 m/s2 in the table of information at the top of the test anyway, so the left side of the equation is completely unneeded.
|
|  | theevilmachines
2009-09-06 20:53:13 | You can easily solve this problem by assuming that the earth is entirely water (most of it is), so the density is 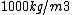 . Then the mass is about . Then the mass is about (6.4 \times 10^6)^3 \approx 10^{24}) . .
pavamanacs
2009-09-16 02:03:34 |
volume of sphere is (4/3) pi r^3 is it not..........
|
justguessing
2009-10-05 10:33:25 |
i like this! he says the Earth is a cube filled with water! that would be so awesome.
|
Albert
2009-11-03 03:15:47 |
Dude! By treating the sphere like a cube, you are off by at least 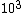 . You are just plain lucky that in the question other options are simply greater than . You are just plain lucky that in the question other options are simply greater than 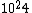 or else you would have been badly confused. This is simply not the best analogy to solve this problem. or else you would have been badly confused. This is simply not the best analogy to solve this problem.
|
Crandor
2010-04-07 22:19:23 |
By neglecting the 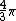 , the exponent is off by , the exponent is off by =0.622) . Before the last approximation, the answer was . Before the last approximation, the answer was \left(6.4\times 10^{6}\right)^{3}=10^{23.419}) . Rounding to 24 took that missing factor into account. Also, the density approximation is good enough for government work. . Rounding to 24 took that missing factor into account. Also, the density approximation is good enough for government work.
|
ashestofeonix
2010-08-31 01:54:18 |
uhh... I hate to be a stickler about something as silly as correct units but.... the density of water is 1 g/cm, that is 1kg/dm^3... And most of the earth is not water... most of the SURFACE of the earth is covered by water, but most of the volume is iron & nickel. There really is a big difference.
|
his dudeness
2010-09-03 19:29:40 |
Not really. Water has a density of 1000 kg/m^3, while Iron has a density of ~8000 kg/m^3. By slightly overestimating the volume of the earth (cube instead of sphere), and slightly underestimating its density, you come reasonably close to the real answer.
|
Almno10
2010-11-12 12:58:34 |
First of all, 4/3*pi will NOT change the factor of ten, which is the only thing you need to get the answer right.
The iron/nickel argument still wont change the factor of ten. 8000 = 8(10^3). Same factor of ten. At most, this will shift your answer by one power of ten...but all the choices are separated by 10^3, so whats the problem? Give the guy a break, cool answer.
|
|  | jax
2005-12-04 20:24:47 | You need to use curly brackets {} around superscripts or else only the first number of your exponent will show up as an exponent!
And I agree that SlickAce21's solution is much simpler. I would not have remembered the mass of the sun, or the density of the earth on the exam!
yosun
2005-12-04 23:17:42 |
jax: thanks for the typo-alert; it's been corrected. SlickAce21: thanks for the alternate solution.
|
|  | SlickAce21
2005-11-11 11:05:34 | This problem is pretty easily solved by a usage of Kepler's law, if one remembers that g = (GM)/R^2, then one gets 10 = (6E-11)M/36E12, multiplying through you have M = 60E23 or 6E24 |  | SlickAce21
2005-11-11 11:03:44 | |  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
