|
GR8677 #14
|
|
|
Problem
|
|
 |
Thermodynamics }Exact differentials }Exact differentials
The key equation is: 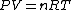 , and its players, , and its players,  , are terms one should be able to guess. , are terms one should be able to guess.
(A) True, according to the ideal gas law. (This is also the final step in deriving Mayer's Equation, as shown below.)
(B) This translates into the statement 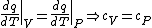 .The problem gives away the fact that for an ideal gas .The problem gives away the fact that for an ideal gas 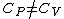 . B can't be right. . B can't be right.
(C) According to the ideal gas law, the volume might change.
(D) False. An ideal gas's internal energy is dependent only on temperature. More elegantly, 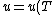) . .
(E) Heat needed for what?
If one is interested in the formal proof of the relation 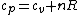 , read on about Mayer's equation: , read on about Mayer's equation:
For thermo, in general, there's an old slacker's pride line that goes like, ``When in doubt, write a bunch of equations of states and mindlessly begin taking exact differentials. Without exerting much brainpower, one will quickly arrive at a brilliant result." Doing this,
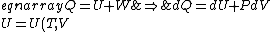&\Rightarrow& dU=\left.\partial_T U\right|_T +\left.\partial_V U\right|_V\\
PV=nRT&\Rightarrow&PdV+VdP=nRdT<br />
\end{eqnarray})
Plugging in the first law of thermodynamics into the 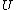 equation of state, one gets equation of state, one gets 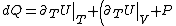dV=\left.\partial_T U\right|_T+PdV) , where the last simplification is made by remembering the fact that the internal energy of an ideal gas depends only on temperature. , where the last simplification is made by remembering the fact that the internal energy of an ideal gas depends only on temperature.
(Taking the derivative with respect to T at constant volume, one gets 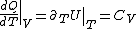 .) .)
Plugging in the simplified result for 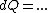 into the third equation of state, the ideal gas equation, one gets: into the third equation of state, the ideal gas equation, one gets:  . Taking the derivative at constant pressure, one gets: . Taking the derivative at constant pressure, one gets:
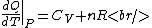
So, one sees that it is the ideal gas equation that makes the final difference. The work of an ideal gas changes when temperature is varied.
|
|
|
Comments |
casseverhart13
2019-09-23 09:41:48 | Sunny Sprinkler Repair |  | ernest21
2019-08-10 03:09:27 | You really just need to look at the limiting factors in this problem. jatt lol |  | fredluis
2019-08-08 13:01:07 | This is the way I understand this problem i really like this solution. tile contractor |  | joshuaprice153
2019-08-08 06:54:34 | Well, this post is quite good!Thanks for updating my information about the subject. It is very useful for me. house painting |  | altheman
2011-08-08 19:53:24 | E can be eliminated because it says that heat needed for constant volume is greater than heat needed to constant pressure which is indicating that c_V > c_P which is the reverse |  | a19grey2
2008-11-02 21:39:18 | There's a free Stat/Mech book online at:
http://stp.clarku.edu/notes/
It certainly isn't a great book, but it's not terrible and it's 100% free. Also, since it's electronic it can be search for keywords which makes it very useful as a resource.
sbrent88
2009-07-09 12:31:55 |
http://stp.clarku.edu/notes/
|
|  | kevglynn
2006-10-31 09:53:04 | I love Stat Mech, and one of the reasons is probably because I love the book that my professor used/wrote for the course. It's awesome, and everyone should check it out:
Elementary Lectures in Statistical Mechanics
by George D. J. Phillies
ISBN: 0387989188 |  | matno
2005-10-31 20:58:48 | What is a good book on Stat Mech???
yosun
2005-11-01 02:30:26 |
The book that I started out with was Classical and Statistical Thermodynamics by A. Carter. On the Stat part, it has a decent introduction to the distributions and the combinatorics involved in deriving them. Further understanding of probability can be gained from Reif. Kittel, Kittel and Kroemer and Ashcroft are also good supplements (three seperate books).
|
yosun
2005-11-01 16:06:25 |
More on Stat Mech textbooks: Sturge offers a good conceptual intro for Bose-Einstein Condensation.
|
Richard
2007-11-02 11:11:00 |
I won't say it's GREAT, but it made for a fun read when I took the course:
An Introduction to Thermal Physics, by Daniel V. Schroeder.
The problems are hellish though.
|
neon37
2008-10-31 00:07:09 |
yea schroeder book is pretty good. I really liked some of his explanations. I took quantum before I took stat mech and I must say that a lot of things in quantum only made sense after I took stat mech.
|
zeus
2012-02-03 09:43:32 |
I'm using Schroeder right now in a statistical mechanics class, and it seems to be a fine book. The writing style is somewhat conversational and straightforward, and the problems show a nice range of difficulty. There are some really basic problems that can be used as a kind of check on your reading, and the harder problems tend to be broken up into smaller, well-guided instructional steps. I would definitely recommend it for self-study.
|
kronotsky
2018-10-23 03:10:02 |
You should buy it for many other reasons, but I\'ve found that \"Modern Classical Physics\" from Thorne and Blandford has the best philosophical explanation of thermodynamics and statistical mechanics I\'ve encountered so far. The treatment really is limited to a basic course, however, so it\'s not what you should get if you want lots of detail (there are only a few chapters on thermal physics!).
|
|  |
|
| Post A Comment! |
|
|
Bare Basic LaTeX Rosetta Stone
|
LaTeX syntax supported through dollar sign wrappers $, ex., $\alpha^2_0$ produces  . .
|
| type this... |
to get... |
| $\int_0^\infty$ |
 |
| $\partial$ |
 |
| $\Rightarrow$ |
 |
| $\ddot{x},\dot{x}$ |
 |
| $\sqrt{z}$ |
 |
| $\langle my \rangle$ |
 |
| $\left( abacadabra \right)_{me}$ |
_{me}) |
| $\vec{E}$ |
 |
| $\frac{a}{b}$ |
 |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
