|
GR0177 #66
|
|
|
|
|
Alternate Solutions |
dipanshugupta
2017-03-31 10:12:40 | Here \\\'s my method, using \\makebf{Picking Numbers}. Pick a time, say 12, because it is divisible both by 24 and 36. In 12 mins, \\alpha decays \\frac{1}{4} and \\beta decays \\frac{1}{6} . Add them up to get \\frac{5}{12} , which is close to half. Take a time little more than 12. Hence, 14.4 minutes. Pick C. |  | travis.nicholson
2006-10-23 01:04:11 | The crux of this problem is that the total decay rate of the sample is equal to the sum of the decay rates through each decay channel.
N = -k_total*N)
Since the half life, , is given by , is given by /\tau)
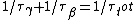
Therefore, 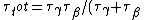 = 14.4min) |  |
|
|
Comments |
dipanshugupta
2017-03-31 10:12:40 | Here \\\'s my method, using \\makebf{Picking Numbers}. Pick a time, say 12, because it is divisible both by 24 and 36. In 12 mins, \\alpha decays \\frac{1}{4} and \\beta decays \\frac{1}{6} . Add them up to get \\frac{5}{12} , which is close to half. Take a time little more than 12. Hence, 14.4 minutes. Pick C. |  | djh101
2014-09-07 23:16:00 | Here's what I did:
It's going to be less than both of the decay rates by themselves, so A and B are out. Then, without wasting too much time, I needed to find a formula that would take two input numbers and give a smaller number. Well, the parallel resistor formula does just that. 14.4, answer C.
djh101
2014-09-07 23:16:47 |
D, sorry.
|
|  | x-rat
2013-10-15 13:59:13 | I think you can solve this without knowing any of the reduced mass/activity. If both 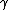 and and 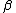 had 24min half life, the total half life would be 12mins. If they both had 36, it would be 18 mins. The only answer in between is 14.4. had 24min half life, the total half life would be 12mins. If they both had 36, it would be 18 mins. The only answer in between is 14.4. |  | Crand0r
2010-11-10 20:42:14 | For something faster and easier to do in your head, find the least common multiple of the half-lives (in this case, 72 min.) and divide by the sum of the number of times each half-life goes into the LCM (3 + 2 = 5). That is, after 72 minutes, the number of molecules will have been halved 3 times through 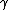 -emission and 2 times through -emission and 2 times through 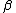 -emission, so it's been halved 5 times, and, thus, that amount of time is 5 half-lives.rnrnThis amounts to the same thing everyone else has done here, but it's easier to do in a timed environment. Of course, if you remember the formula off the top of your head, just go with that. -emission, so it's been halved 5 times, and, thus, that amount of time is 5 half-lives.rnrnThis amounts to the same thing everyone else has done here, but it's easier to do in a timed environment. Of course, if you remember the formula off the top of your head, just go with that. |  | anmuhich
2009-03-16 13:11:24 | You can also figure out the answer just by looking at the answers and thinking about rates of decay. If you only had gamma emission half the sample would decay in 24 minutes, so A is out. It's going to be less than 24 minutes because you also have beta emission, so B is out. Since the beta emission is slower than the gamma you know that the half life will be longer than half of the gamma emission. This eliminates E. 20.8 minutes is too close to 24 for that to be the half life of just gamma emissions, especially when you also have the beta emissions, so the answer must be 14.4 D. |  | carl_the_sagan
2008-11-07 20:29:54 | This is just a glorified rate problem like you see on the general GRE. Gamma gets you half the work done in 24 minutes, Beta gets you half the work done in 36 minutes. Working together you have:
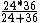 = 14.4 = 14.4
Granted they are really decays, but the same idea applies, and you didn't have to mess with logs and any other nonsense.
giga17
2010-08-11 02:19:21 |
Agree with this. In fact, it is essentially related to the concept of reduced mass.
|
|  | chrisfizzix
2008-09-23 13:38:26 | Here's how I solved this problem, which is the same method except a slightly different form.
We have a decay process with two different channels; the probability that the sample has not decayed at time  from the decay process from the decay process 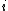 is is 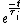 , where , where  is the half-life. The total probability then is is the half-life. The total probability then is  } ) . Then we can find the half-life . Then we can find the half-life 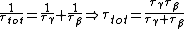 . .
When I was taking this test as practice, I didn't remember anything about decay processes except the simple exponential decay rule, and that probabilities multiply. |  | Blake7
2007-09-21 20:56:10 | Is it just me, or did ETS throw easier numbers at us to work with in 0177?
Come on, divide 24(36)/60 !?!? 14.4 about falls out!
(Oooooh, pardon the pun! Get it? "falls out")
irishroogie
2007-10-02 13:39:56 |
Did you know that an overusage (and particularly a delight in the usage) of puns is a sign of mental illness. Totally random fact but I heard it on the radio and then checked out the literature on it... It's true!!!
(I am not implying anything here Blake7 but be careful :) )
|
|  | travis.nicholson
2006-10-23 01:04:11 | The crux of this problem is that the total decay rate of the sample is equal to the sum of the decay rates through each decay channel.
N = -k_total*N)
Since the half life, , is given by , is given by /\tau)
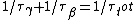
Therefore, 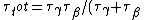 = 14.4min)
natec
2013-09-18 09:46:12 |
anyone else notice that this is exactly analogous to adding resistances in parallel since the two processes are happening in parallel? same idea, same equation.
|
|  | herrphysik
2006-09-28 00:10:45 | Everywhere you have }) you should have you should have }) . You're forgetting the minus sign, and . You're forgetting the minus sign, and }=ln{(2)}) . Plus, remember the simple half-life formula is just . Plus, remember the simple half-life formula is just }/\lambda) . This error doesn't change the answer since it all cancels out, but it adds to the confusion. Also, to answer ee7klt, . This error doesn't change the answer since it all cancels out, but it adds to the confusion. Also, to answer ee7klt, 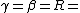 the number of radioactive particles in the sample. the number of radioactive particles in the sample. |  | ee7klt
2005-11-11 04:18:09 | Hi,
How did the 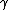 and and 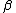 factor out in to factor out in to 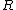 (what is (what is 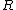 ?) in the second to last step? ?) in the second to last step?
cheers. |  |
|
|
|
|
The Sidebar Chatbox...
Scroll to see it, or resize your browser to ignore it... |
|
|
|
